- Descriptive Vs. Inferential Statistics
- Types of Measurement Scales
- Parameter, Sample Statistic, and Frequency Distribution
- Relative Frequencies and Cumulative Relative Frequencies
- Properties of a Data Set (Histogram / Frequency Polygon)
- Measures of Central Tendency
- Calculating Arithmetic Mean
- Calculating Weighted Average Mean
- Calculating Geometric Mean
- Calculating Harmonic Mean
- Calculating Median and Mode of a Data Set
- Quartiles, Quintiles, Deciles, and Percentiles
- Range and Mean Absolute Deviation
- Variance and Standard Deviation
- Chebyshev’s Inequality
- Coefficient of Variation
- Sharpe Ratio
- Skewness and Kurtosis
- Relative Locations of Mean, Median and Mode
Relative Frequencies and Cumulative Relative Frequencies
The data in a frequency distribution can also be presented using relative frequencies.
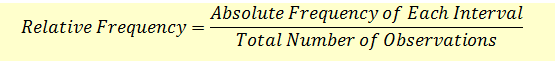
Once we have relative frequencies, we can calculate cumulative relative frequencies where as we move from first frequency interval to the last, we keep adding the relative frequencies finally reaching 100%. Cumulative relative frequencies are useful in measuring what fraction of total observations are less than the upper limit of a frequency interval.
We will extend our example to show the relative frequencies and cumulative relative frequencies.
| Interval | Absolute Frequency | Relative Frequencies | Cumulative Relative Frequencies |
| 0 <= r < 2 | 3 | 3/20 = 15% | 15% |
| 2 <= r < 4 | 5 | 5/20 = 25% | 40% |
| 4 <= r < 6 | 6 | 6/20 = 30% | 70% |
| 6 <= r < 8 | 2 | 2/20 = 10% | 80% |
| 8 <= r < 10 | 4 | 4/20 = 20% | 100% |
| 20 | 100% |
The cumulative relative frequency is equal to the some of the relative frequencies of all the previous intervals including the current interval. For example, the cumulative absolute frequency for the interval 4 <= r < 6 is 15% + 25% + 30% = 70%.
This content is for paid members only.
Join our membership for lifelong unlimited access to all our data science learning content and resources.