Tests Concerning Differences in Means
Sometimes we want to test if the mean values differ between two populations. We can assume that the two populations are normally distributed and that the samples are drawn independently.
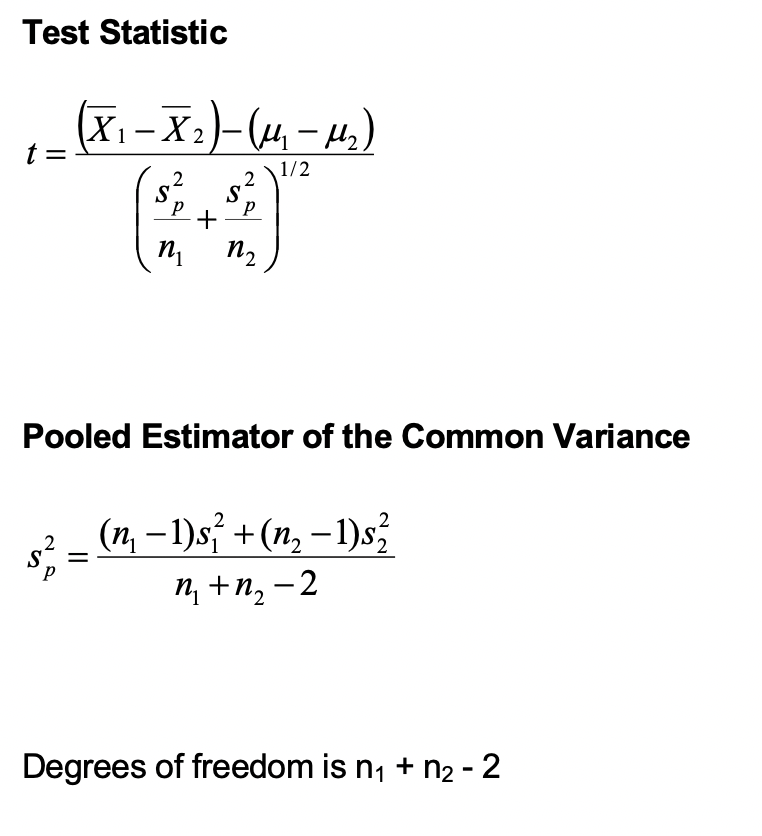
We can combine observations from both samples to get a pooled estimate of the unknown population variance.
The hypothesis can be formed as follows:
- H0: µ1 - µ2 = 0 versus HA: µ1 - µ2 ≠ 0
- H0: µ1 - µ2 ≤ 0 versus HA: µ1 - µ2 > 0
- H0: µ1 - µ2 ≥ 0 versus HA: µ1 - µ2 < 0
Case 1: Normally distributed populations, population variances unknown, but assumed to be equal
Case 2: Normally distributed populations, population variances unequal and unknown



